odd permutations|Odd and Even Permutations

odd permutations,An odd permutation is a permutation obtainable from an odd number of two-element swaps, i.e., a permutation with permutation symbol equal to -1.Any one permutation will consist of either an even number of swaps or an odd number no matter how that is done. An even permutation is one that requires and even number of "swaps", an odd permutation is one that requires an odd .

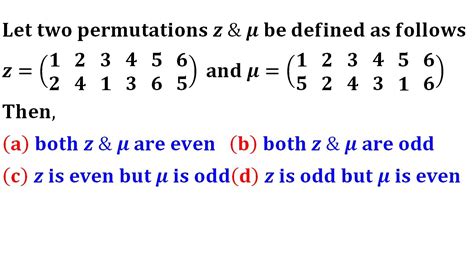
A permutation is called odd if it can be expressed as a product of odd number of transpositions. Example-1: Here we can see that the permutation ( 3 4 5 6 ) has been .In mathematics, when X is a finite set with at least two elements, the permutations of X (i.e. the bijective functions from X to X) fall into two classes of equal size: the even permutations and the odd permutations. If any total ordering of X is fixed, the parity (oddness or evenness) of a permutation of X can be defined as the parity of the number of inversions for σ, i.e., of pairs of elements x, y of X such .This means that when a permutation is written as a product of disjoint cycles, it is an even permutation if the number of cycles of even length is even, and it is an odd permutation if the . We show how to determine if a permutation written explicitly as a product of cycles is odd or even. We call \(\pi\) an even permutation if \(\mbox{sign}(\pi) = +1\), whereas \(\pi\) is called an odd permutation if \(\mbox{sign}(\pi) = -1\). This video explains how to determine if a permutation in cycle notation is even or odd. In this video we explore how permutations can be written as products of 2-cycles, and how this gives rise to the notion of an even or an odd permutation .more. To prove the final assertion, let \(B_n\) be the set of odd permutations and let \(\tau = (1,2)\text{.}\) Define \(\theta: A_n \to B_n\) by \(\theta(f) = f\circ \tau\text{.}\) Suppose .Consider X as a finite set of at least two elements then permutations of X can be divided into two category of equal size: even permutation and odd permutation. Odd Permutation. Odd permutation is a set of permutations obtained from odd number of two element swaps in a set. It is denoted by a permutation sumbol of -1. For a set of n numbers . Solution to (b): The definition of permutation indicates “.no two elements in each list are the same.” Hence the permutation formula cannot be used. However, the rule of products still applies. We have any one of five .

odd permutations Odd and Even Permutations Even and Odd Permutations 3 Groups of Symmetries Linear Isometries The Dihedral groups Symmetry Groups of the Platonic Solids 4 Cayley’s theorem | every group is a permutation group. Abstract Algebra, Lecture 5 Jan Snellman The Symmetric group De nition Conjugation Permutations Groups of Symmetries Cayley’s theorem

Odd permutations map to the non-trivial element $1$ in this map, while even permutations map to the trivial element $0$. This is the reason behind calling them odd and even , because we have the following operations:

Odd permutations map to the non-trivial element $1$ in this map, while even permutations map to the trivial element $0$. This is the reason behind calling them odd and even , because we have the following operations:

The other argument : If we have two odd permutations, the number of transpositions of the product is modulo $2$ equal to the sum of the numbers of transpositions of the permutations. If we have two odd permutations, the product is therefore even because the sum of two odd numbers is even.Every permutation can be written as a product of transpositions; for instance, the permutation g from above can be written as g = (1 2)(2 5)(3 4). Since g can be written as a product of an odd number of transpositions, it is then called an odd permutation, whereas f .

A permutation of \(n\) distinct objects is just a listing of the objects in some order. For example, \([c,b,a]\) is a permutation of the set \(\{a,b,c\}\) of three objects. Likewise, [triangle, melon, airplane] is a permutation of three objects as well. From our mathematical point of view, the objects we use don't actually matter; all we care .further denote by lemaσand lomaσthe numbers of even and odd left-to-right maxima. Similarly we define remiσand romiσto be the numbers of even and odd right-to-left mnima. Let X 2n be the set of permutations in S 2n that contain only even-odd descents. A permutation in X 2n is called an even-odd descent permutation. For n= 1,2, we haveCount the number of possible permutations (ordered arrangement) of n items taken r at a time. Count the number of possible permutations when there are conditions imposed on the arrangements. Prerequisite Skills. Before you get started, take this prerequisite quiz. 1. How many three-letter word sequences can be formed using the letters { A, B, C .事實上在大學的代數中我們學 2-cycle decomposition 只是為了方便去定義什麼是 even permutation 和 odd permutation 罷了. 我們稱 S n 中的元素是 even 如果它可以寫成偶數個 2-cycle 的乘積, 反之則稱為 odd. 你應該會覺得這個定義有點奇怪吧! Let's begin with the observation that, assuming that identity permutation is not an odd permutation, then the result follows fairly easily. Theorem: Assuming the identity permutation is not an odd permutation, then all permutations are either even xor odd. Proof: Let $\sigma$ be both an even and an odd permutation. Then there exists .

Groups, Even and Odd Permutations Even and Odd Permutations . A permutation of n elements can be written as an ordered list of the numbers 1 through n. (Other notational conventions exist; this one will be familiar to computer programmers.) For instance, the permutation x = {2,3,1,5,4} moves the element in position 1 to position 2, 2 to 3, and . A transposition is clearly an odd permutation. Composing a permutation with a single transposition alters the parity of the number of inversions. The product of two even permutations, and also of two odd ones, is an even permutation, while the product of an even and an odd permutation (in either order) is odd.odd permutations In this video we explain even and Odd Permutations.A Permutation is even if it can be written in the product of even number of transpositions.This video inc.Every permutation in \(S_n\) can be expressed as a product of 2-cycles (Transpositions). The number of transpositions will either always be even or odd. If a permutation α can be expressed as a product of an even number of 2-cycles, then every decomposition of α into a product of 2-cycles has an even number of 2-cycles. A similar statement .Odd and Even Permutations The grand total is therefore $$1+3+6+0+1+3+1+0+0= 15\;,$$ if I’ve not miscounted. We conclude that the permutation $259148637$ has $15$ inversions, and since $15$ is odd, it’s an odd permutation. Finding the inverse is another matter altogether.

The map sign that assigns to every permutation its signature is a group homomorphism from the symmetric group to the multiplicative group $\{−1, 1\}$: $$ \text{sign}: S_n \to \{−1, 1\}. $$ By definition, a permutation is even if its signature is $1$ and a permutation is is odd if its signature is $-1$.

Hence the permutation is odd. There are many ways to write a permutation as the product of transpositions; but the number of transpositions required to do so will always be even, or always be odd. So one can prove "odd-ness" (respectively "even-ness") by expressing the permutation as the product of transpositions, using your favorite method for .

odd permutations|Odd and Even Permutations

odd permutations|Odd and Even Permutations .

Share:

×

Share

Copy Link

Email

Facebook

Twitter

LinkedIn

WhatsApp

Download: Full Size (80225 MB)

Photo By: odd permutations|Odd and Even Permutations

VIRIN: 44523-50786-27744